Square area
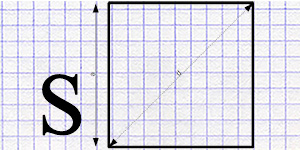
The square — is a quadrilateral that possesses a number of unique characteristics, making it one of the most studied and applied geometric figures. A square is defined as a regular polygon with four equal sides and four 90-degree angles. This definition emphasizes two main properties of the square: the equality of all its sides and right angles.
The properties of the square make it an object with a high degree of symmetry. The square has several types of symmetry: axial symmetry relative to its diagonals, which are also equal to each other and intersect at a 90-degree angle in the center of the figure, and central symmetry relative to the point of intersection of the diagonals. Moreover, a square can be described around a circle and inscribed in a circle, making it the only rectangle with this property.
These fundamental properties of the square underlie its geometric characteristics and determine the methods for calculating the area and other parameters. Understanding these properties allows for a deeper study and application of concepts related to squares in various mathematical and practical tasks.
Formula for the Area of a Square
The area of a square — is a measure of the space bounded by its sides. There are several ways to calculate the area of a square, depending on the available data: the length of the side, the length of the diagonal, the radius of the inscribed or circumscribed circle.
1. Through the side of the square (\(a\))
The area of a square can be calculated if the length of its side is known. The formula for the area of a square through the side is expressed as:
\[S = a^2\]
where \(S\) — is the area of the square, \(a\) — is the length of the square's side.
2. Through the diagonal of the square (\(d\))
If the length of the square's diagonal is known, the area can be found using the following formula:
\[S = \frac{d^2}{2}\]
This formula is based on the Pythagorean theorem, where the diagonal divides the square into two isosceles right triangles, and the length of the diagonal is the hypotenuse of these triangles.
3. Through the radius of the inscribed circle (\(r\))
The radius of the inscribed circle is related to the area of the square as follows:
\[S = 4r^2\]
This follows from the fact that the radius of the inscribed circle is equal to half the length of the square's side.
4. Through the radius of the circumscribed circle (\(R\))
The area of the square can also be calculated through the radius of the circumscribed circle:
\[S = 2R^2\]
This formula is based on the relationship between the radius of the circumscribed circle and the length of the diagonal of the square, where \(R\) is equal to half the length of the diagonal.
Each of these methods allows finding the area of the square depending on the available data, providing flexibility in solving geometric tasks.
Examples of Calculating the Area of a Square
For a better understanding of the application of square area formulas, let's consider several practical examples:
1. Calculating the area through the length of the side
Assume the side of the square \(a\) is equal to 5 meters. Using the formula \(S = a^2\), we find the area of the square:
\[S = 5^2 = 25\ \text{m}^2\]
Thus, the area of a square with a side of 5 meters is 25 square meters.
2. Calculating the area through the length of the diagonal
If the length of the square's diagonal \(d\) is known to be 7 meters, then the area of the square can be found using the formula \(S = \frac{d^2}{2}\):
\[S = \frac{7^2}{2} = \frac{49}{2} = 24.5\ \text{m}^2\]
Therefore, a square with a diagonal of 7 meters has an area of 24.5 square meters.
3. Calculating the area through the radius of the inscribed circle
Suppose the radius of the inscribed circle \(r\) is 2 meters. Then the area of the square is calculated as \(S = 4r^2\):
\[S = 4 \times 2^2 = 4 \times 4 = 16\ \text{m}^2\]
Thus, a square with an inscribed circle radius of 2 meters has an area of 16 square meters.
4. Calculating the area through the radius of the circumscribed circle
If the radius of the circumscribed circle \(R\) is equal to 4 meters, the area of the square is found using the formula \(S = 2R^2\):
\[S = 2 \times 4^2 = 2 \times 16 = 32\ \text{m}^2\]
A square circumscribed around a circle with a radius of 4 meters has an area of 32 square meters.
These examples demonstrate various ways to calculate the area of a square, making mathematical formulas universal tools for solving tasks in the field of geometry.
Tasks and Exercises for Independent Solution
To consolidate the material and develop skills in solving geometric problems, we offer the following tasks on the topic "Area of a Square":
1. Basic tasks for calculating the area
- Task 1: The length of the side of the square is 6 cm. Find the area of the square.
- Task 2: The area of the square is 49 sq.m. Determine the length of the side of the square.
2. Tasks for calculating the area through the diagonal
- Task 3: The diagonal of the square is 10 cm. Calculate the area of the square.
- Task 4: The area of the square is 50 sq.cm. Find the length of its diagonal.
3. Tasks on the application of the radius of the inscribed and circumscribed circle
- Task 5: The radius of the circle inscribed in the square is 3 cm. Find the area of the square.
- Task 6: The radius of the circle circumscribed around the square is \(5\sqrt{2}\) cm. Determine the area of the square.
4. Tasks of increased complexity
- Task 7: A square is inscribed in a circle with a radius of 4 cm. Find the area of the square.
- Task 8: A circle is inscribed in a square with a side of 8 cm. Find the radius of this circle and the area of the square.
After attempting to solve the tasks independently, check your answers by applying the formulas for the area of a square through known parameters. This exercise will help not only to consolidate knowledge on the topic but also to develop the ability to apply theoretical knowledge in practice, as well as improve problem-solving skills.
Comments on the calculator
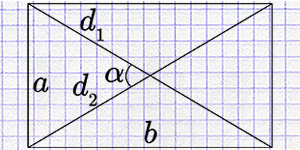
Calculator for computing rectangle area by two sides, diagonal, angle, and radius of the circumscribed circle, among various other methods.
Go to calculation
The calculator allows calculating the area of a circle based on its radius, diameter, or circumference in mm, cm, or meters.
Go to calculation
Calculator for calculating the surface area of the human body based on height and weight values.
Go to calculation