Fraction addition and subtraction calculator
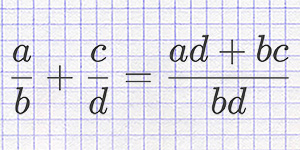
Mastering the addition and subtraction of fractions is necessary not only for solving mathematical problems but also for developing logical thinking, the ability to analyze and synthesize information, which is an integral part of the educational process in school and an important skill in everyday life. We will consider different types of fractions: proper, improper, and mixed, and show how this knowledge is applied to perform mathematical operations of addition and subtraction. This knowledge will open up endless possibilities for solving a wide range of problems, from simple arithmetic to complex engineering calculations, and help improve your mathematical literacy.
Fundamentals of Fractions
The fundamentals of fractions lay the foundation for understanding the addition and subtraction of fractions, as well as other mathematical operations with them. A fraction represents a part of a whole or the ratio between two numbers and consists of two components: the numerator and the denominator. The numerator indicates the number of equal parts, and the denominator indicates how many parts the whole is divided into. Depending on the ratio of the numerator to the denominator, fractions can be proper, improper, and mixed.
- Proper fractions have a numerator smaller than the denominator, meaning they represent a part smaller than the whole. An example of a proper fraction is \( \frac{3}{4} \).
- Improper fractions have a numerator larger than the denominator or equal to it, meaning they represent a whole or a part that exceeds the whole. An example of an improper fraction is \( \frac{5}{3} \).
- Mixed numbers consist of a whole number and a proper fraction, which allows for a more visual representation of the number. An example of a mixed number is \( 1\frac{2}{3} \).
For successful addition and subtraction of fractions, it is important to be able to bring them to a common denominator, which allows operations on fractions with different denominators. This is achieved by finding the least common denominator (LCD), which is the least common multiple of the denominators of the given fractions.
Mastering these fundamentals of fractions is necessary for further study of mathematics and successful completion of more complex tasks, including the addition and subtraction of fractions, their multiplication and division, and their application in practical situations where working with parts of a whole is required.
Addition of Fractions
Addition of fractions is a mathematical operation that allows combining two or more fractions into one sum. The process of adding fractions depends on whether the fractions have the same denominators or different ones. To successfully perform the addition of fractions, it is important to follow certain rules.
Adding Fractions with the Same Denominators
Adding fractions with the same denominators is the simplest case. In this case, it is enough to add the numerators of the fractions, while leaving the denominator unchanged. The result of the addition will be a fraction with the same denominator and the sum of the numerators of the original fractions.
Example: \( \frac{3}{5} + \frac{2}{5} = \frac{3 + 2}{5} = \frac{5}{5} = 1 \).
Adding Fractions with Different Denominators
To add fractions with different denominators, it is necessary to bring them to a common denominator. This is achieved by finding the least common denominator (LCD) for the denominators of the original fractions and bringing the fractions to an equivalent form with this common denominator.
After bringing the fractions to a common denominator, the numerators are added similarly to the case with the same denominators, while the denominator remains common.
Example: \( \frac{1}{3} + \frac{2}{4} \). The LCD for 3 and 4 is 12, so we bring the fractions to the form \( \frac{4}{12} + \frac{6}{12} = \frac{10}{12} \). Then, the fraction can be simplified to \( \frac{5}{6} \).
Practical Tips
- Always simplify the fraction to the lowest possible expression after addition.
- Use the least common denominator to simplify calculations and achieve a more accurate result.
Adding fractions requires attention to details and understanding of the basic principles of working with fractions. Mastering these skills will ensure successful completion of both simple and more complex tasks involving fractions.
Subtraction of Fractions
Subtraction of fractions is a process similar to addition, but instead of adding values, the numerators of the fractions are subtracted from each other. As in the case of addition, the process of subtraction depends on whether the fractions have the same denominators or different ones.
Subtracting Fractions with the Same Denominators
When fractions have the same denominators, subtraction is performed by subtracting the numerators, while the denominator remains unchanged. This is the simplest case, requiring minimal effort to perform.
Example: \( \frac{5}{7} - \frac{2}{7} = \frac{5 - 2}{7} = \frac{3}{7} \).
Subtracting Fractions with Different Denominators
To subtract fractions with different denominators, you first need to find a common denominator for both fractions. This process is similar to finding a common denominator when adding fractions and includes finding the Least Common Denominator (LCD) for the denominators of both fractions.
Once the fractions are expressed with a common denominator, their numerators are subtracted from one another, while the common denominator remains unchanged.
Example: \( \frac{3}{4} - \frac{1}{6} \). The LCD for 4 and 6 is 12, so we convert the fractions to \( \frac{9}{12} - \frac{2}{12} = \frac{7}{12} \).
Practical Tips
- Always check if the fraction obtained after subtraction can be simplified to a simpler expression.
- Use the least common denominator to simplify the process and increase the accuracy of calculations.
Mastering the skills of subtracting fractions will ease solving many mathematical problems and deepen the understanding of working with fractional numbers. Practice and attention to detail will help you successfully tackle this task.
Simplifying Fractions After Addition and Subtraction
Simplifying fractions after addition and subtraction is a key step that helps make the answer more understandable and easier for further calculations. This process involves reducing the fraction to its simplest possible expression, where the numerator and denominator of the fraction are divided by their Greatest Common Divisor (GCD).
Finding the GCD
To simplify a fraction, you first need to find the greatest common divisor of the numerator and denominator. The GCD is the largest number that both the numerator and denominator can be divided by without a remainder.
Example of Simplification
Consider the fraction \( \frac{8}{12} \). The GCD for 8 and 12 is 4. Dividing both the numerator and denominator by 4, we get the simplified fraction \( \frac{2}{3} \).
Why It's Important to Simplify Fractions
- Simplified fractions are easier to understand and use in subsequent calculations.
- It standardizes answers, making them comparable and convenient for verification.
- Simplifying fractions helps visualize and analyze mathematical models and equations.
Using Simplified Fractions
After simplifying a fraction, it can be used in more complex mathematical operations, such as multiplying and dividing fractions, as well as in tasks involving ratios and percentages. A simplified fraction can also serve as the final answer, presented in the most compact and understandable form.
In conclusion, simplifying fractions after addition and subtraction is an integral part of working with fractions, making it easier to understand and use mathematical expressions in various fields of knowledge.
Common Mistakes in Adding and Subtracting Fractions
When working with adding and subtracting fractions, common mistakes often occur that can lead to incorrect results. Understanding and avoiding these mistakes will help improve your math skills and increase the accuracy of your calculations.
Ignoring the Need for a Common Denominator
One of the most common mistakes is attempting to add or subtract fractions with different denominators without first converting them to a common denominator. Always ensure that you have found a common denominator before performing the operation.
Incorrect Simplification of Fractions
After adding or subtracting fractions, it is important to correctly simplify the result. A mistake may occur if the greatest common divisor for the numerator and denominator is not found, leading to an answer that could be further simplified.
Incorrect Addition of Numerators and Denominators
Some students mistakenly add or subtract the numerators and denominators as separate numbers, leading to incorrect results. Remember that addition and subtraction operations are only performed with the numerators, while the denominator remains common for the result.
Forgetting to Reduce Fractions
After obtaining the answer, it is important to check if the fraction can be further simplified. Skipping this step leaves the answer in an unsimplified form, which may not be as easy to interpret or use in further calculations.
Errors in Calculations
Simple arithmetic errors in calculating the numerator or denominator can lead to incorrect final results. It is always useful to double-check your calculations.
Avoiding these common mistakes requires practice and attention to detail. Over time and with experience, you will become quicker at noticing and correcting these errors, improving your skills in working with fractions.
Comments on the calculator
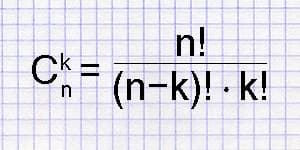
The combinations calculator helps you determine the count of potential combinations from a specified number of objects, denoted as "n choose k."
Go to calculation
The service will help factorize any composite number into its prime factors with a step-by-step solution.
Go to calculation
A Bernoulli trial is a random experiment with two possible outcomes, typically labeled as "success" and "failure".
Go to calculation